atm20-100

decomposition variable_bound set_partitioning invariant_knapsack mixed_binary

Submitter	Variables	Constraints	Density	Status	Group	Objective	MPS File
Matthew Galati	6480	4380	2.07445e-03	hard	–	2463621.57727541	atm20-100.mps.gz

ATM cash management problem. Solved with COIN/DIP in 1066 sec. (1 thread) / 259 sec. (8 threads) in June 2011. Imported from MIPLIB2010.

Location of instance in collection

Instance Statistics

Detailed explanation of the following tables can be found here.

Size Related Properties
	Original	Presolved
Variables	6480	6469
Constraints	4380	4369
Binaries	2220	2209
Integers	0	0
Continuous	4260	4260
Implicit Integers	0	0
Fixed Variables	0	0
Nonzero Density	0.00207445	0.00208205
Nonzeroes	58878	58845

Constraint Classification Properties
	Original	Presolved
Total	4380	4369
Empty	0	0
Free	0	0
Singleton	0	0
Aggregations	0	0
Precedence	0	0
Variable Bound	2220	2209
Set Partitioning	20	20
Set Packing	0	0
Set Covering	0	0
Cardinality	0	0
Invariant Knapsack	20	20
Equation Knapsack	0	0
Bin Packing	0	0
Knapsack	0	0
Integer Knapsack	0	0
Mixed Binary	2120	2120
General Linear	0	0
Indicator	0	0

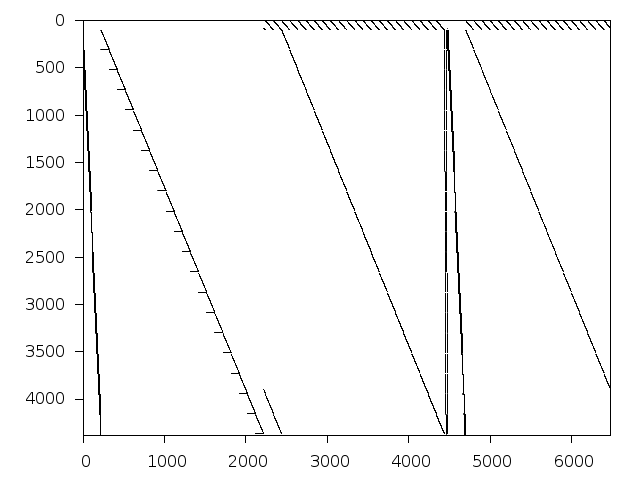
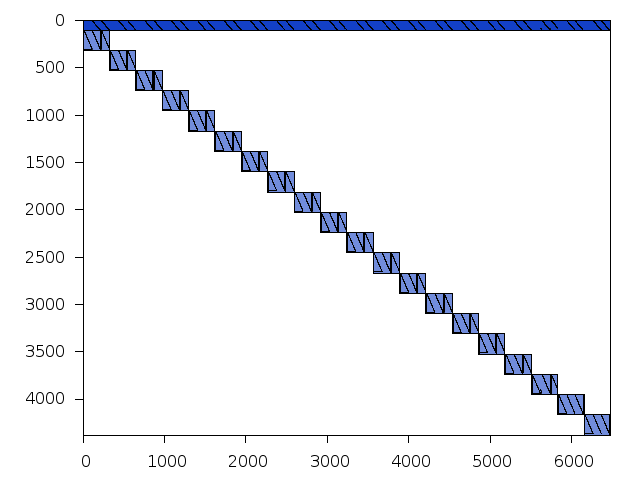
Structure

Available nonzero structure and decomposition information. Further information can be found here.

Nonzero entries of original problem

Nonzero entries after trivial presolving

Decomposed structure of original problem (dec-file)

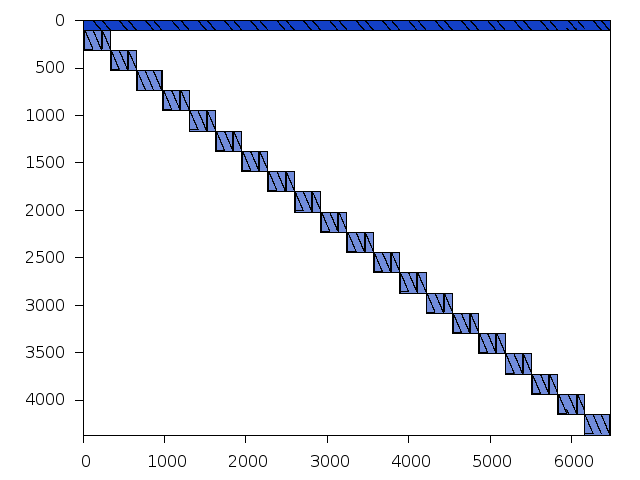
Decomposed structure after trivial presolving (dec-file)

	value	min	median	mean	max
Components	1.322219
Constraint %		4.82948	4.88556	4.89815	4.89815
Variable %		4.95370	4.99151	5.00000	5.00000
Score	0.928338

Best Known Solution(s)

No solution available for atm20-100 .

Similar instances in collection

The following instances are most similar to atm20-100 in the collection. This similarity analysis is based on 100 scaled instance features describing properties of the variables, objective function, bounds, constraints, and right hand sides.

Instance	Status	Variables	Binaries	Continuous	Constraints	Nonz.	Submitter	Group	Objective	Tags
mtest4ma	easy	1950	975	975	1174	4875	MIPLIB submission pool	–	52148	decomposition variable_bound set_packing mixed_binary
australia-abs-cta	easy	49758	918	48840	3946	33792	Jordi Castro	cta	106.0589096028744	decomposition numerics aggregations variable_bound mixed_binary
sp150x300d	easy	600	300	300	450	1200	MIPLIB submission pool	sp_product	69	benchmark decomposition benchmark_suitable aggregations variable_bound mixed_binary
lotsize	easy	2985	1195	1790	1920	6565	Dinakar Gade, Simge Kucukyavuz	–	1480195	benchmark decomposition benchmark_suitable variable_bound set_packing invariant_knapsack mixed_binary
danoint	easy	521	56	465	664	3232	Daniel Bienstock	dano	65.6666666666	benchmark_suitable variable_bound cardinality mixed_binary

Reference

@phdthesis{Galati2010,
 author = {Matthew Galati},
 language = {English},
 school = {Lehigh University},
 title = {Decomposition Methods for Integer Linear Programming},
 year = {2010}
}