bley_xs2
numerics aggregations variable_bound set_partitioning set_packing set_covering invariant_knapsack knapsack mixed_binary general_linear
| Submitter | Variables | Constraints | Density | Status | Group | Objective | MPS File |
|---|---|---|---|---|---|---|---|
| A. Bley | 2515 | 2257 | 3.67507e-03 | easy | bley_x | 1051266.38 | bley_xs2.mps.gz |
Min-cost network dimensioning problem with finite sets of link capacities and unsplittable flow routing Imported from the MIPLIB2010 submissions.
Instance Statistics
Detailed explanation of the following tables can be found here.
| Original | Presolved | |
|---|---|---|
| Variables | 2515 | 2373 |
| Constraints | 2257 | 1833 |
| Binaries | 1632 | 1580 |
| Integers | 883 | 793 |
| Continuous | 0 | 0 |
| Implicit Integers | 0 | 0 |
| Fixed Variables | 0 | 0 |
| Nonzero Density | 0.00367507 | 0.00415752 |
| Nonzeroes | 20861 | 18084 |
| Original | Presolved | |
|---|---|---|
| Total | 2257 | 1833 |
| Empty | 19 | 0 |
| Free | 0 | 0 |
| Singleton | 54 | 0 |
| Aggregations | 53 | 52 |
| Precedence | 0 | 0 |
| Variable Bound | 30 | 5 |
| Set Partitioning | 45 | 45 |
| Set Packing | 0 | 191 |
| Set Covering | 89 | 99 |
| Cardinality | 0 | 0 |
| Invariant Knapsack | 0 | 32 |
| Equation Knapsack | 0 | 0 |
| Bin Packing | 0 | 0 |
| Knapsack | 318 | 184 |
| Integer Knapsack | 0 | 0 |
| Mixed Binary | 403 | 56 |
| General Linear | 1246 | 1169 |
| Indicator | 0 | 0 |
Structure
Available nonzero structure and decomposition information. Further information can be found here.
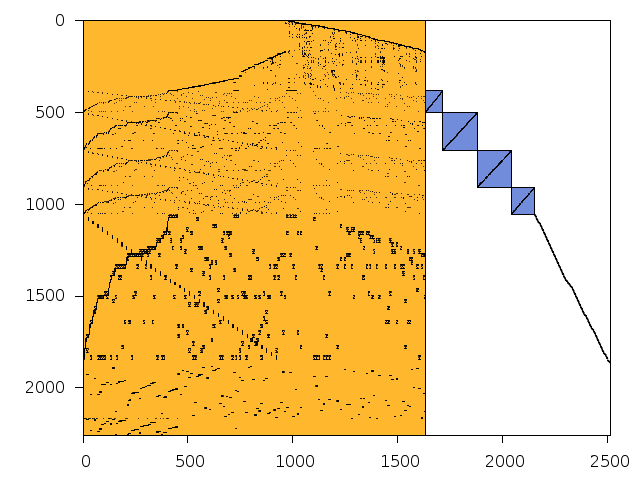
Decomposed structure of original problem (dec-file)

Decomposed structure after trivial presolving (dec-file)
| value | min | median | mean | max | |
|---|---|---|---|---|---|
| Components | 2.348305 | ||||
| Constraint % | 0.0545554 | 0.104687 | 0.0545554 | 7.80142 | |
| Variable % | 0.0824742 | 0.294418 | 0.2474230 | 6.80412 | |
| Score | 0.226580 |
Best Known Solution(s)
Find solutions below. Download the archive containing all solutions from the Download page.
| ID | Objective | Exact | Int. Viol | Cons. Viol | Obj. Viol | Submitter | Date | Description |
|---|---|---|---|---|---|---|---|---|
| 1 | 1051266 | 1051266 | 0 | 0 | 0 | - | 2018-10-11 | Solution found during MIPLIB2017 problem selection. |
Similar instances in collection
The following instances are most similar to bley_xs2 in the collection. This similarity analysis is based on 100 scaled instance features describing properties of the variables, objective function, bounds, constraints, and right hand sides.
Reference
No bibliographic information availableLast Update 2024 by Julian Manns
generated with R Markdown
© by Zuse Institute Berlin (ZIB)
Imprint