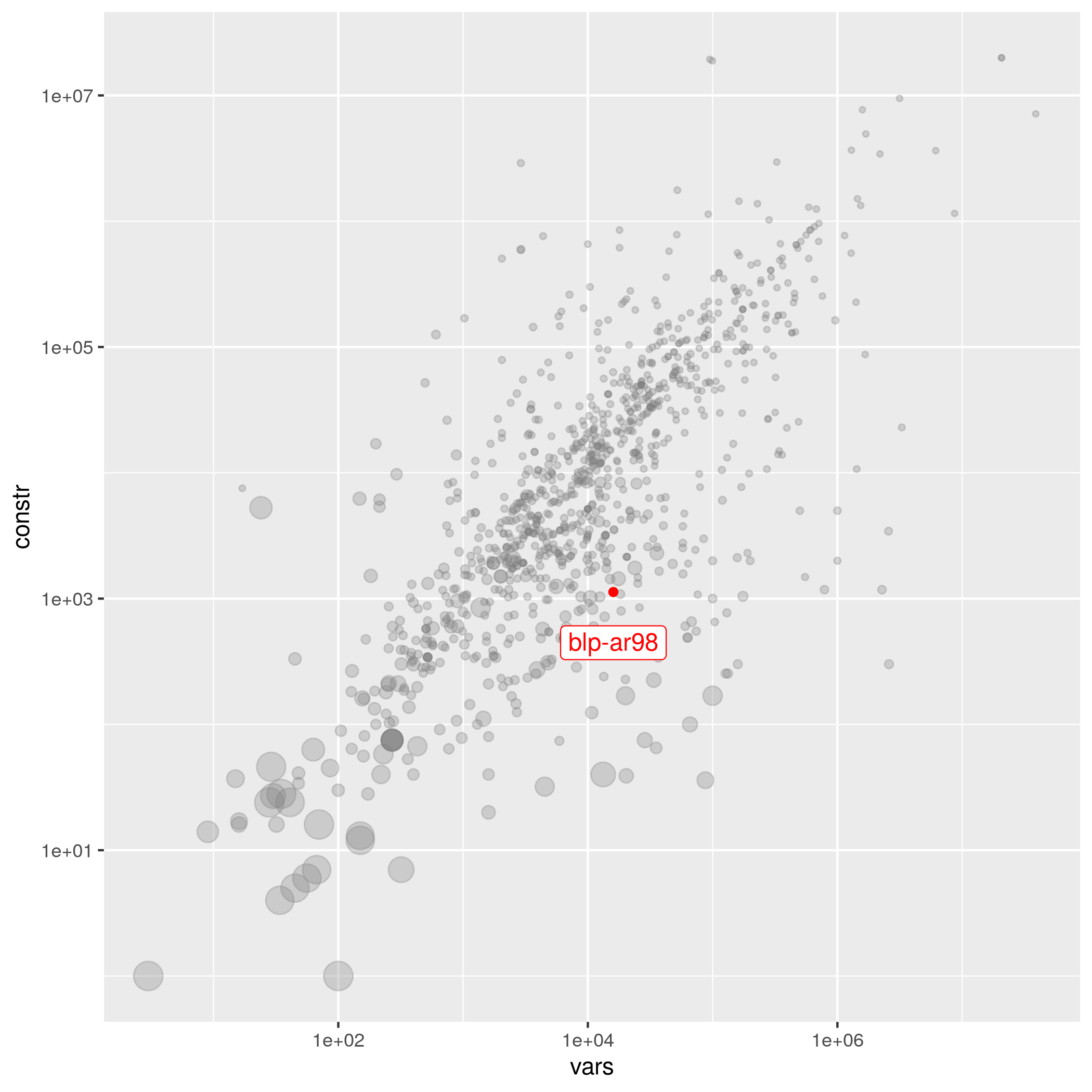
blp-ar98

benchmark decomposition benchmark_suitable variable_bound set_packing equation_knapsack mixed_binary general_linear

Submitter	Variables	Constraints	Density	Status	Group	Objective	MPS File
M. Lübbecke	16021	1128	1.11003e-02	easy	blp	6205.2147104	blp-ar98.mps.gz

Railway line planning instance Imported from MIPLIB2010.

Location of instance in collection

Instance Statistics

Detailed explanation of the following tables can be found here.

Size Related Properties
	Original	Presolved
Variables	16021	16017
Constraints	1128	1128
Binaries	15806	15806
Integers	0	210
Continuous	215	1
Implicit Integers	0	210
Fixed Variables	4	0
Nonzero Density	0.0111003	0.0111028
Nonzeroes	200601	200597

Constraint Classification Properties
	Original	Presolved
Total	1128	1128
Empty	0	0
Free	0	0
Singleton	0	0
Aggregations	0	0
Precedence	0	0
Variable Bound	1	1
Set Partitioning	0	0
Set Packing	912	912
Set Covering	0	0
Cardinality	0	0
Invariant Knapsack	0	0
Equation Knapsack	0	4
Bin Packing	0	0
Knapsack	0	0
Integer Knapsack	0	0
Mixed Binary	215	1
General Linear	0	210
Indicator	0	0

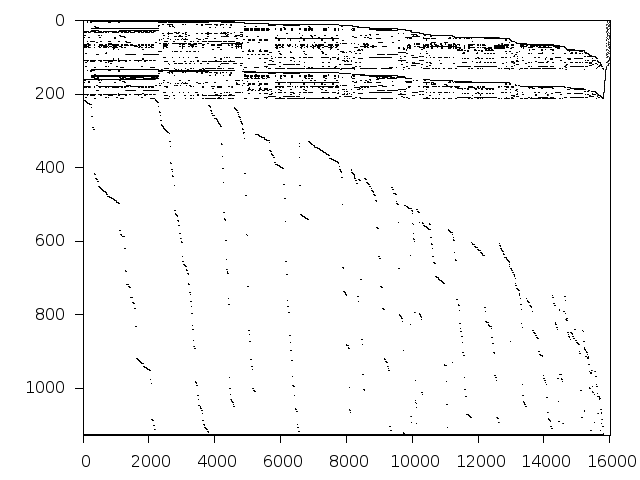
Structure

Available nonzero structure and decomposition information. Further information can be found here.

Nonzero entries of original problem

Nonzero entries after trivial presolving

Decomposed structure of original problem (dec-file)

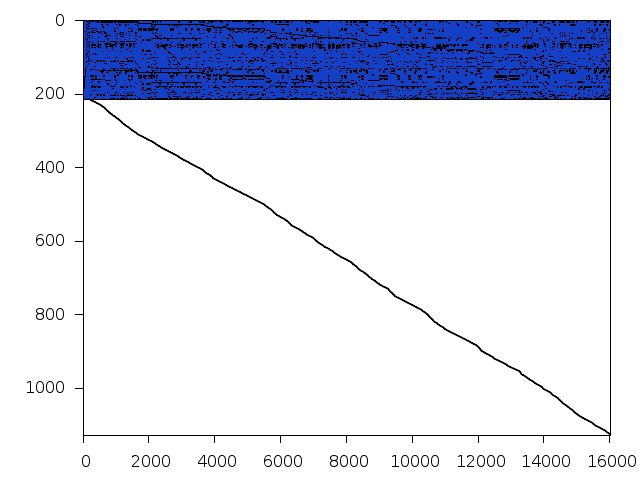
Decomposed structure after trivial presolving (dec-file)

	value	min	median	mean	max
Components	2.960946
Constraint %		0.0886525	0.0886525	0.0886525	0.0886525
Variable %		0.0124836	0.1080590	0.1248360	0.1560450
Score	0.808523

Best Known Solution(s)

Find solutions below. Download the archive containing all solutions from the Download page.

ID	Objective	Exact	Int. Viol	Cons. Viol	Obj. Viol	Submitter	Date	Description
2	6205.215	6205.215	0	0	0	-	2018-10-13	Solution imported from MIPLIB2010.
1	6205.215	6205.215	0	0	0	-	2018-10-13	Solution found during MIPLIB2017 problem selection.

Similar instances in collection

The following instances are most similar to blp-ar98 in the collection. This similarity analysis is based on 100 scaled instance features describing properties of the variables, objective function, bounds, constraints, and right hand sides.

Instance	Status	Variables	Binaries	Continuous	Constraints	Nonz.	Submitter	Group	Objective	Tags
blp-ir98	easy	6097	6031	66	486	79152	M. Lübbecke	blp	2342.315488	decomposition benchmark_suitable set_packing equation_knapsack mixed_binary general_linear
blp-ic98	easy	13640	13550	90	717	191947	M. Lübbecke	blp	4491.44758395	benchmark decomposition benchmark_suitable set_packing mixed_binary general_linear
blp-ic97	easy	9845	9753	92	923	118149	M. Lübbecke	blp	4025.023580799999	decomposition benchmark_suitable set_packing mixed_binary general_linear
neos-3116779-oban	easy	5141	5140	1	328	20561	Jeff Linderoth	neos-pseudoapplication-26	0	decomposition set_packing knapsack general_linear
leo1	easy	6731	6730	1	593	131218	COR@L test set	–	404227536.16	benchmark benchmark_suitable variable_bound set_packing set_covering mixed_binary

Reference

@article{BussieckLindnerLuebbecke2004,
 author = {M. R. Bussieck and T. Lindner and M. E. L{\"u}bbecke},
 journal = {Mathematical Methods of Operations Research},
 number = {2},
 pages = {205-220},
 title = {A Fast Algorithm for Near Optimal Line Plans},
 volume = {59},
 year = {2004}
}

@article{FischettiGloverLodi2005,
 author = {M. Fischetti and F. Glover and A. Lodi},
 journal = {Mathematical Programming},
 pages = {91--104},
 title = {The feasibility pump},
 volume = {104},
 year = {2005}
}