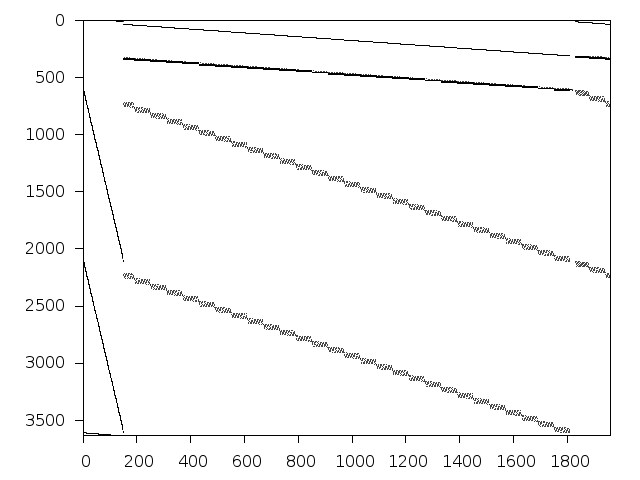
control30-5-10-4

infeasible decomposition numerics variable_bound set_partitioning mixed_binary

Submitter	Variables	Constraints	Density	Status	Group	Objective	MPS File
Qie He	1960	3640	3.00796e-03	easy	control	Infeasible	control30-5-10-4.mps.gz

Optimal control of a discrete-time switched system model Although the underlying mathematical model has a feasible solution by construction, most solvers report this instance as infeasible and/or unbounded.

Location of instance in collection

Instance Statistics

Detailed explanation of the following tables can be found here.

Size Related Properties
	Original	Presolved
Variables	1960	1950
Constraints	3640	3630
Binaries	150	150
Integers	0	0
Continuous	1810	1800
Implicit Integers	0	0
Fixed Variables	0	0
Nonzero Density	0.00300796	0.00302889
Nonzeroes	21460	21440

Constraint Classification Properties
	Original	Presolved
Total	3640	3630
Empty	0	0
Free	0	0
Singleton	10	0
Aggregations	0	0
Precedence	0	0
Variable Bound	3000	3000
Set Partitioning	30	30
Set Packing	0	0
Set Covering	0	0
Cardinality	0	0
Invariant Knapsack	0	0
Equation Knapsack	0	0
Bin Packing	0	0
Knapsack	0	0
Integer Knapsack	0	0
Mixed Binary	600	600
General Linear	0	0
Indicator	0	0

Structure

Available nonzero structure and decomposition information. Further information can be found here.

Nonzero entries of original problem

Nonzero entries after trivial presolving

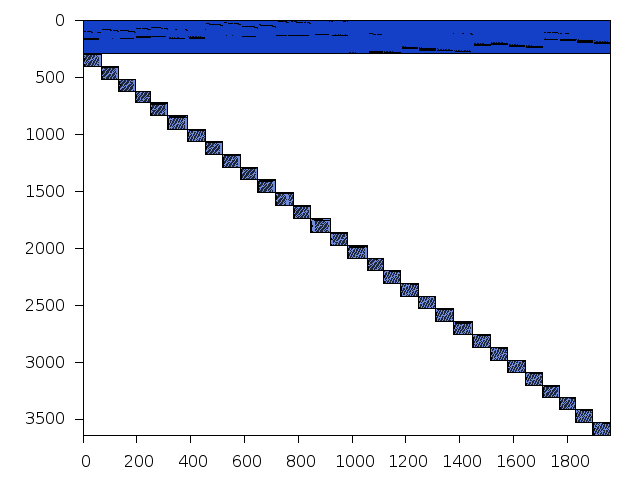
Decomposed structure of original problem (dec-file)

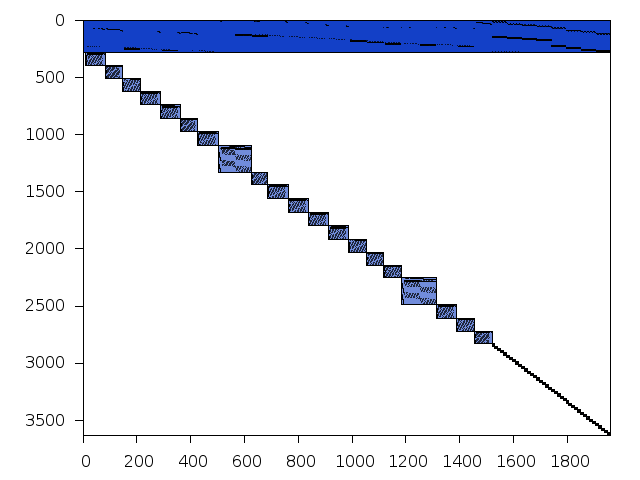
Decomposed structure after trivial presolving (dec-file)

	value	min	median	mean	max
Components	1.491362
Constraint %		3.05785	3.06703	3.05785	3.33333
Variable %		3.31633	3.31633	3.31633	3.31633
Score	0.889596

Best Known Solution(s)

No solution available for control30-5-10-4 .

Similar instances in collection

The following instances are most similar to control30-5-10-4 in the collection. This similarity analysis is based on 100 scaled instance features describing properties of the variables, objective function, bounds, constraints, and right hand sides.

Instance	Status	Variables	Binaries	Continuous	Constraints	Nonz.	Submitter	Group	Objective	Tags
control20-5-10-5	easy	1310	100	1210	2430	14710	Qie He	control	406205618311810	decomposition numerics variable_bound set_partitioning mixed_binary
control30-3-2-3	easy	332	90	242	512	1472	Qie He	control	-17.25658927192	decomposition numerics variable_bound set_partitioning mixed_binary
neos-799711	easy	41998	910	41088	59218	147164	NEOS Server Submission	neos-pseudoapplication-25	-11170211.734	numerics aggregations precedence variable_bound set_partitioning set_packing cardinality mixed_binary
rentacar	easy	9557	55	9502	6803	41842	MIPLIB submission pool	–	30356760.98379315	aggregations precedence variable_bound mixed_binary
npmv07	easy	220686	1880	218806	76342	859614	Q. Chen	–	104809812543.8479	numerics aggregations variable_bound mixed_binary

Reference

@TECHREPORT{HeWu2017, 
author="Q. He and Z. Wu", 
title="Optimal control of a discrete-time switched system", 
INSTITUTION ={Department of Industrial and Systems Engineering, University of Minnesota},
month={February},
year="2017"}