fastxgemm-n3r21s3t6

decomposition variable_bound set_partitioning set_covering mixed_binary general_linear

Submitter	Variables	Constraints	Density	Status	Group	Objective	MPS File
Laurent Sorber	18684	219368	1.75214e-04	open	fastxgemm	4110.99800117485*	fastxgemm-n3r21s3t6.mps.gz

Naive multiplication of two N by N matrices requires N^3 scalar multiplications. For N=2, Strassen showed that it could be done in only R=7 < 8=N^3 multiplications. For N=3, it is known that 19 <= R <= 23, and for N=4 it is known that 34 <= R <= 49. This repository contains code that generates a mixed-integer linear program (MILP) formulation of the fast matrix multiplication problem for finding solutions with R < N^3 and proving that they are optimal. For a more detailed description, see the accompanying manuscript.

Location of instance in collection

Instance Statistics

Detailed explanation of the following tables can be found here.

Size Related Properties
	Original	Presolved
Variables	18684	18684
Constraints	219368	219368
Binaries	378	378
Integers	0	1134
Continuous	18306	17172
Implicit Integers	0	1134
Fixed Variables	0	0
Nonzero Density	0.000175214	0.000175214
Nonzeroes	718146	718146

Constraint Classification Properties
	Original	Presolved
Total	219368	219368
Empty	0	0
Free	0	0
Singleton	0	0
Aggregations	0	0
Precedence	46494	0
Variable Bound	46494	92988
Set Partitioning	0	567
Set Packing	0	0
Set Covering	0	90
Cardinality	0	0
Invariant Knapsack	0	0
Equation Knapsack	0	0
Bin Packing	0	0
Knapsack	0	0
Integer Knapsack	0	0
Mixed Binary	126380	2684
General Linear	0	123039
Indicator	0	0

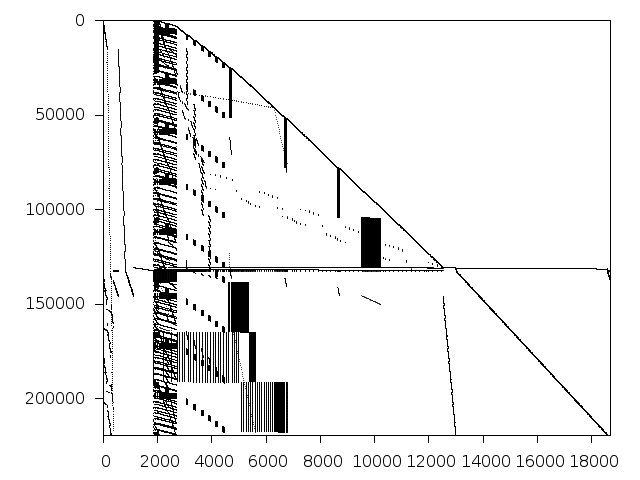
Structure

Available nonzero structure and decomposition information. Further information can be found here.

Nonzero entries of original problem

Nonzero entries after trivial presolving

Decomposed structure of original problem (dec-file)

Decomposed structure after trivial presolving (dec-file)

	value	min	median	mean	max
Components	1.342423
Constraint %		4.67707	4.67707	4.67707	4.67707
Variable %		4.33526	4.33526	4.33526	4.33526
Score	0.939605

Best Known Solution(s)

Find solutions below. Download the archive containing all solutions from the Download page.

## Warning in lapply(df["exactobjval"], as.numeric): NAs introduced by coercion

ID	Objective	Exact	Cons. Viol	Submitter	Date	Description
4	4110.998		3e-07	Edward Rothberg	2019-12-13	Obtained with Gurobi 9.0
3	6084.000	6084	0e+00	Frederic Didier	2020-01-22	Obtained with Google OR-tools using 8 Threads through generating subproblems by fixing part of the current solution and trying to solve them with a sub CP-SAT solver
2	9084.000		0e+00	Robert Ashford and Alkis Vazacopoulus	2019-12-18	Found using ODH\|CPlex
1	21084.000	21084	0e+00	-	2018-10-13	Solution found during MIPLIB2017 problem selection.

Similar instances in collection

The following instances are most similar to fastxgemm-n3r21s3t6 in the collection. This similarity analysis is based on 100 scaled instance features describing properties of the variables, objective function, bounds, constraints, and right hand sides.

Instance	Status	Variables	Binaries	Integers	Continuous	Constraints	Nonz.	Submitter	Group	Objective	Tags
fastxgemm-n3r22s4t6	open	19539	396	0	19143	229742	752274	Laurent Sorber	fastxgemm	3101.998498499999*	decomposition variable_bound set_partitioning set_covering mixed_binary general_linear
fastxgemm-n3r23s5t6	open	20394	414	0	19980	240116	786402	Laurent Sorber	fastxgemm	3089.997998*	decomposition variable_bound set_partitioning set_covering mixed_binary general_linear
fastxgemm-n2r7s4t1	easy	904	56	0	848	6972	22584	Laurent Sorber	fastxgemm	41.99999999999998	decomposition benchmark_suitable variable_bound set_partitioning set_covering mixed_binary general_linear
fastxgemm-n2r6s0t2	easy	784	48	0	736	5998	19376	Laurent Sorber	fastxgemm	230	benchmark decomposition benchmark_suitable variable_bound set_partitioning set_covering mixed_binary general_linear
neos-4335793-snake	hard	30827	20473	7865	2489	37166	129119	Jeff Linderoth	neos-pseudoapplication-44	27	numerics aggregations precedence variable_bound set_packing cardinality invariant_knapsack knapsack mixed_binary general_linear

Reference

@misc{Sorber2017,
author = {Laurent Sorber and Marc Van Barel},
title = {{A mixed-integer linear program formulation for fast matrix multiplication}},
howpublished = "\url{https://github.com/lsorber/fast-matrix-multiplication/blob/master/latex/fast-matrix-multiplication.pdf}",
day = {30},
month = {April},
year = {2017}, 
note = "[Online]"
}