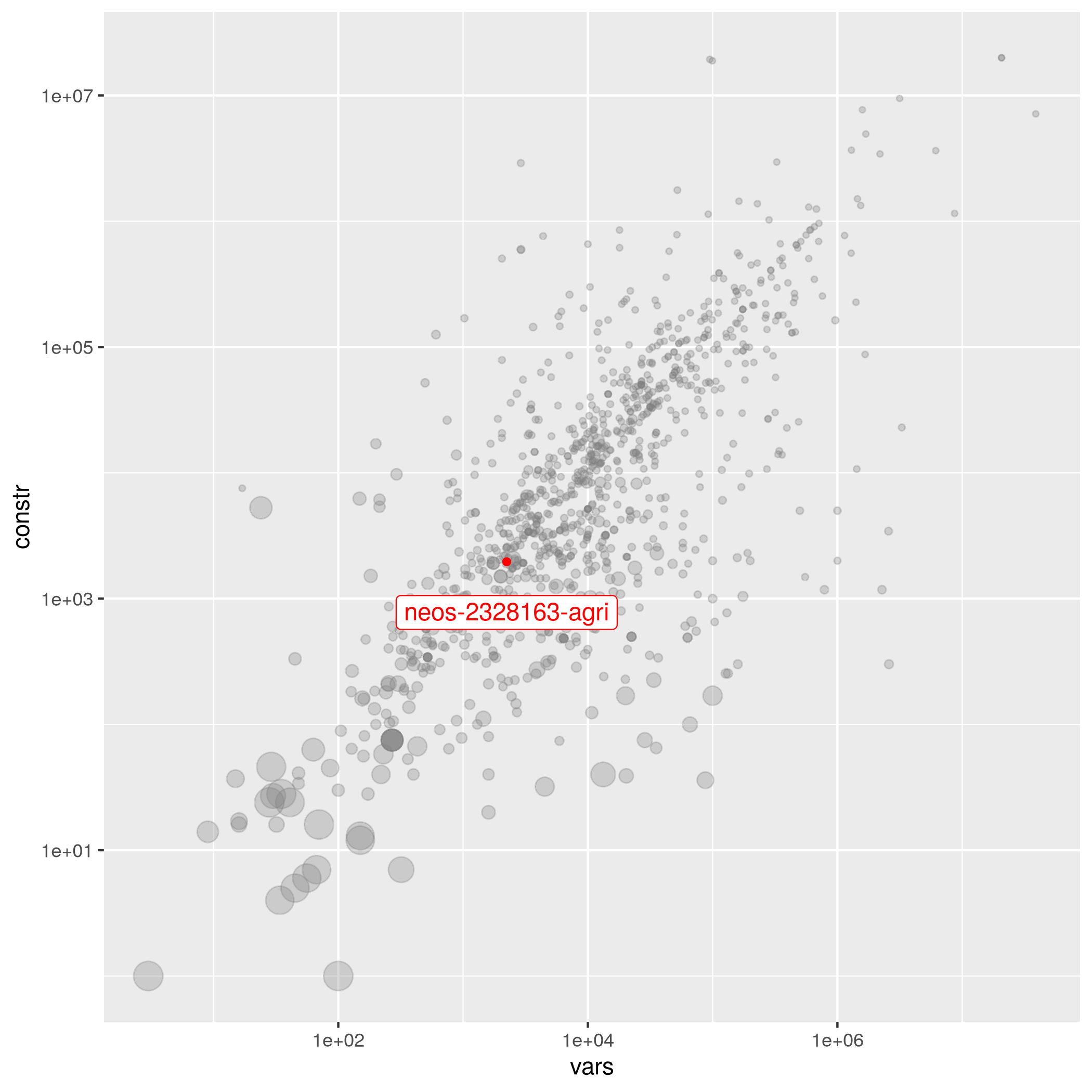
neos-2328163-agri

binary decomposition benchmark_suitable set_partitioning set_packing set_covering cardinality invariant_knapsack binpacking knapsack

Submitter	Variables	Constraints	Density	Status	Group	Objective	MPS File
Jeff Linderoth	2236	1963	2.90253e-03	easy	neos-pseudoapplication-36	27674	neos-2328163-agri.mps.gz

Location of instance in collection

Instance Statistics

Detailed explanation of the following tables can be found here.

Size Related Properties
	Original	Presolved
Variables	2236	2236
Constraints	1963	1963
Binaries	2236	2236
Integers	0	0
Continuous	0	0
Implicit Integers	0	0
Fixed Variables	0	0
Nonzero Density	0.00290253	0.00290253
Nonzeroes	12740	12740

Constraint Classification Properties
	Original	Presolved
Total	1963	1963
Empty	0	0
Free	0	0
Singleton	0	0
Aggregations	0	0
Precedence	0	0
Variable Bound	0	0
Set Partitioning	104	104
Set Packing	104	104
Set Covering	52	52
Cardinality	13	13
Invariant Knapsack	78	78
Equation Knapsack	0	0
Bin Packing	0	1560
Knapsack	0	52
Integer Knapsack	0	0
Mixed Binary	1612	0
General Linear	0	0
Indicator	0	0

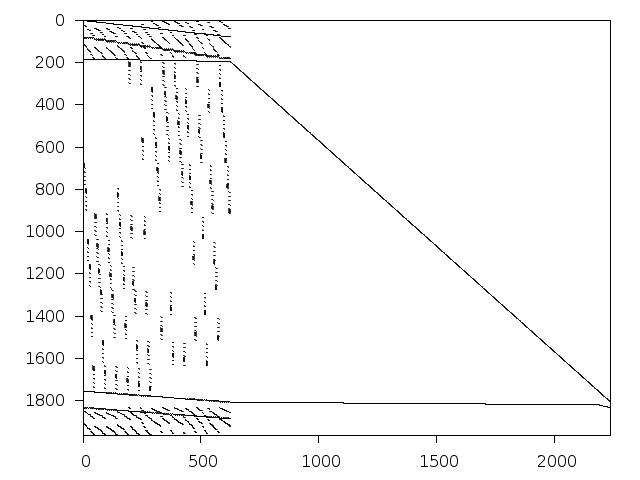
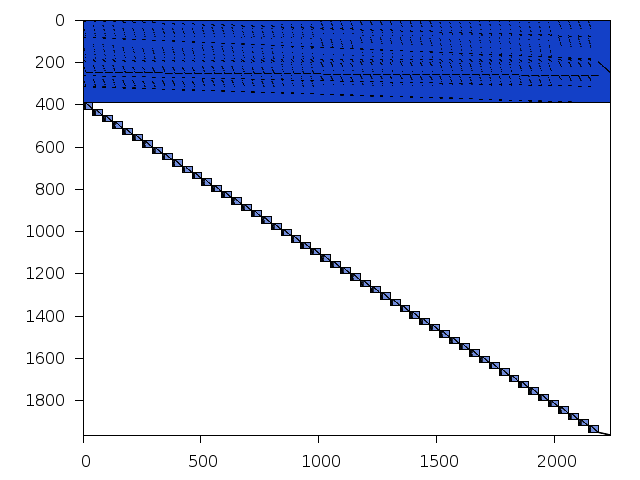
Structure

Available nonzero structure and decomposition information. Further information can be found here.

Nonzero entries of original problem

Nonzero entries after trivial presolving

Decomposed structure of original problem (dec-file)

Decomposed structure after trivial presolving (dec-file)

	value	min	median	mean	max
Components	1.819544
Constraint %		0.0519751	1.25780	1.55925	1.55925
Variable %		0.1788910	1.53846	1.87835	1.87835
Score	0.802326

Best Known Solution(s)

Find solutions below. Download the archive containing all solutions from the Download page.

ID	Objective	Exact	Int. Viol	Cons. Viol	Obj. Viol	Submitter	Date	Description
1	27674	27674	0	0	0	-	2018-10-15	Solution found during MIPLIB2017 problem selection.

Similar instances in collection

The following instances are most similar to neos-2328163-agri in the collection. This similarity analysis is based on 100 scaled instance features describing properties of the variables, objective function, bounds, constraints, and right hand sides.

Instance	Status	Variables	Binaries	Continuous	Constraints	Nonz.	Submitter	Group	Objective	Tags
peg-solitaire-a3	easy	4552	4552	0	4587	28387	Hiroshige Dan ; Koichi Fujii	pegsolitaire	1	benchmark binary benchmark_suitable aggregations variable_bound set_partitioning cardinality binpacking
neos-4333464-siret	easy	2731	1363	1368	2880	27710	Jeff Linderoth	neos-pseudoapplication-58	24.78581995155556	benchmark_suitable variable_bound binpacking mixed_binary
neos-4343293-stony	hard	9400	4698	4702	10650	55668	Jeff Linderoth	neos-pseudoapplication-58	46.38468271624112	variable_bound binpacking mixed_binary
neos-4355351-swalm	open	21065	10530	10535	21609	371467	Jeff Linderoth	neos-pseudoapplication-58	33.45757454008309*	variable_bound binpacking mixed_binary
neos-4387871-tavua	hard	4004	2000	2004	4554	23496	Jeff Linderoth	neos-pseudoapplication-58	33.384729927	benchmark benchmark_suitable variable_bound binpacking mixed_binary

Reference

No bibliographic information available